(1) Volume equivalent
The amount of differential pressure due to leaks depends on the internal volume of the measuring system as a whole, including WORK and the tester. This also strongly related to measurement time.
With air leak testers, we use as this internal volume a coefficient called volume equivalent (Ve), which accounts for the effects of causes for pressure change throughout the measuring system as a whole (volumetric changes due to the internal pressures of WORK or the differential pressure sensor).
The volume equivalent can be calculated using the following equation.
Depending on the model of air leak tester, the auto leak calibrator (ALC) or the leak MASTER can be used to measure the value of Ve directly.
Ve=Vw+Vt+{Ks(1+Vw/Vm)+Kw}(101.3+P)
Vw=Vm (WORK and MASTER are same in volume)
Ks=0.01 mL / kPa or 0.005 mL/kPa (depending on model)
Kw=0mL/kPa (no change in WORK volume)
Under conditions above
Ks(1+Vw/Vm)+Kw=2Ks
| When Ks = 0.01 mL / kPa
Ve=Vw+Vt+0.02(101.3+P)
| When Ks = 0.005 mL / kPa
Ve=Vw+Vt+0.01(101.3+P)
Ve: Volume equivalent (mL)
Vw:Internal volume of WORK and tubing (mL)
Vt: Internal volume of tester (mL) Vt = 9 mL
Ks:The ratio of volumetric change of the sensor per pressure unit (mL / kPa)
Kw: The ratio of volumetric change of WORK per pressure unit
P:Test pressure (kPa)
(2) Leak rate
The relational expression for volume equivalent and leak rate Q
| Q :Leak rate(mL/min) ΔP:Differential pressure(Pa) Ve:Volume equivalent (mL) T:Detection time(s) |
Example: A differential pressure of 15 Pa was measured when the volume equivalent was 800 mL and the detection time was 3 seconds. What is the leak rate under these conditions? 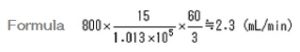
| <※COMMENT> The differential pressure which occurs due to leaks is inversely proportional to the volume equivalent. [Example] When the ratio of the volume equivalent is 1 to 5, the differential pressure at the same leak rate would be 5 to 1. Q:5mL/min T:2s ΔP1 = 85 Pa when Ve = 200 mL ΔP2 = 17 Pa when Ve = 1000 mL | 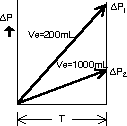 |
<Reference>Pressure and characteristics of gases
| a. Absolute pressure: Pressure with absolute vacuum as its reference point E.g. PaA, kg/cm2A (or kg/cm2abs), Torr (absolute pressure = atmospheric pressure + gauge pressure) b. Gauge pressure: Pressure with atmospheric pressure as its reference point (positive pressure/vacuum pressure) E.g. PaG, kg/cm2G c. Differential pressure: the difference between two pressures e.g. PaD, kg/cm2D | 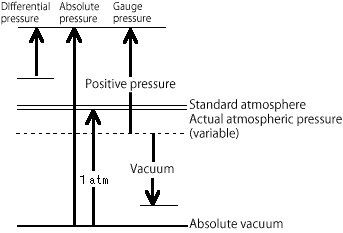 |

2) Units of pressure 1 bar = 0.1 MPa = 1.01972 kg/cm2, 1 Pa = 1 N/m2, 1 Torr = 1 mmHg
| Pa | kg/cm2 | mmH2O | mmHg | PSI | |
| 1Pa | 1 | 1.01972×10E-5 | 0.101972 | 7.50062×10E-3 | 1.45038×10E-4 |
| 1kg/cm2 | 9.80665×10E4 | 1 | 10E4 | 735.559 | 14.2233 |
| 1mmH2O | 9.80665 | 10E-4 | 1 | 7.35559×10E-2 | 1.42233×10E-3 |
| 1mmHg | 133.322 | 1.35951×10E-3 | 13.5951 | 1 | 1.93368×10E-2 |
| 1PSI | 6894.76 | 7.03070×10E-2 | 703.070 | 51.7149 | 1 |
Note: “10E-3” indicates 10 to the negative third power
(3)Equation of state of gases
There is a constant relationship among three quantities of pressure, volumetric capacity, and temperature of gases. Once two of them are determined, the other is consequently determined.
The equation describing the relationship among these three quantities is called an equation of state, consisting of the “combined gas law.”
PV=GRT…………(1)
Initial state Final state
When (V1, P1, T1) → (V2, P2, T2) 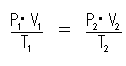 If the temperature is constant T1 = T2, P1⋅V1 = P2⋅V2 ……… (2)
If the temperature is constant T1 = T2, P1⋅V1 = P2⋅V2 ……… (2)
If the volumetric capacity is constant V1 = V2, P1 / T1 = P2 / T2 ……… (3)
P:Absolute pressure
V: Volumetric capacity
G:Weight of gas
T:Absolute temperature
R:Gas constant
(1)Detection time setting
The differential pressure value and detection time (T3) corresponding to the leak standard value Q are found and set using the volume equivalent Ve.Settings of a detection time of 2 to 10 seconds and a differential pressure value ΔP in the range of ±10 to 100 Pa are generally suitable.
| Q :Leak rate (mL/min) Ve :Volume equivalent (mL) ΔP:Differential pressure (Pa) T :Detection time (s) If using a leak tester with the leak rate direct-read display feature, there is no need to set the differential pressure. |
(2)Guidelines for finding pressurization and stabilization time:
For Intelligent 1 Pneumatic Circuit(LS-1822A、LS-1862)
Pressurization time (CHG) to Stabilization time (BAL) = 3 to 1 or 4 to 1
For Intelligent 2 Pneumatic Circuit(LS-1881、LS-1842)
Pressurization time (CHG) to Stabilization1 (BAL1) to Stabilization2 (BAL2) = 5 to 5 to 1
Note: When WORK volumetric capacity is low, pressure equalizing time is not required. Set BAL1 to 0 seconds.
These times usually depend on the condition of the actual machinery, equipment, and tooling, as well as the characteristics of WORK, and therefore must be found by experimentation.
They can also be estimated for WORK which have been used in the past.
Required pressurization and stabilization time (Pressurization time CHG + Stabilization BAL) ->
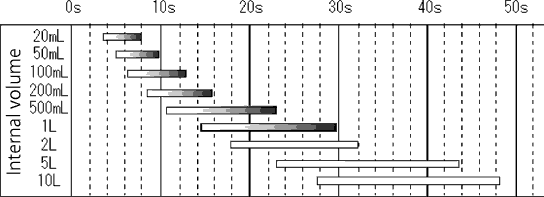
※The above graph does not include detection time.
※Time can also change depending on the precision required.
※Low pressure <- Test pressure -> High pressure ![]() Low pressure (10–100kPa) High pressure (100–600kPa)
Low pressure (10–100kPa) High pressure (100–600kPa)
<※COMMENT>
When setting the measurement time while performing experiments or startup adjustments, confirm first that there are definitely no leaks in WORK or the toolings.Then, start with ample pressurization time to obtain data.Gradually shorten this time and find the shortest pressurization and stabilization times where data is stable around zero.
(1)Comparison of leak rates of gases and liquids in practice
In general, the leak rate of air and liquids (water, oil) is determined according to the Hagen–Poiseuille law, by their viscosity and pressure.However, an assumption is that the leak hole is a round pipe with a smooth surface.In practice, leaks from bubbles in cast metal and pinholes from defective welding have rough, irregular surfaces with bumps and bends.Theoretical equations do not necessarily apply to the correlation of gases and liquids in such cases.
A phenomenon occurs with minute leaks—air may leak, but liquids may not leak. In most cases of minute leaks, the ratio of the leak rates of air and liquids are much greater than in theory. This is thought to be because surface tension works on the liquid and the inner surface of the leak hole, increasing flow resistance.
<※COMMENT>
※Cautions when performing experiments comparing leak flow
Often, the leak rates of gases and liquids do not correlate due to foreign matter. Especially when using air to measure a leak hole which has already leaked liquid, the leak rate could become smaller or stop, making reproducibility difficult to obtain. When performing experiments comparing leak flow, make sure to test with air first.
<※COMMENT>
Hagen–Poiseuille equation
- Symbols
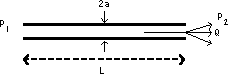
- Leak rate: Q [atm mL/s]
Atmospheric pressure: P0 = 1.013 × 105 [Pa abs]
Small IN tube pressure: P1 [Pa abs]
Small OUT tube pressure: P2 [Pa abs]
Average pressure: ‾P [Pa abs]
Hole diameter: 2 a [m]
Hole length: L [m]
Fluid viscosity coefficient: η [Pa·s]
1 Pa・s = 1 kg/m・s = 1 N・s/m2 = 1000 cP = 10 Poise or g/cm・s
(2)Theoretical equations comparing leak rates of gases and liquids
Ql:Liquid leak rate(atm mL/s) ηa:Air viscosity(Pa・s) ηl:Liquid viscosity(Pa・s) Pa:Air Test pressure(kPa) Pl:Liquid test pressure(kPa) |  |
<※COMMENT>
If the test pressure has been changed for the same gas, the new leak rate can be found using the following equation. ![]() Equation for leak rate
Equation for leak rate
P1:Test pressure before change (kPa)
P2:Test pressure after change (kPa)
Q1:Leak rate before change (mL/min)
Q2:Leak rate after change (mL/min)
Leak rate ratio will differ from the theoretical equation in cases where there is a possibility of a change in the leak hole shape when the test pressure is changed, e.g. leaks in gasket seals.
To express the differential pressure in terms of leak rate, the volume equivalent must be measured. This is called leak calibration, a factor essential for a leak tester1. Measurement of volumetric equivalent (Ve) using a volumetric-change-method leak calibrator.
Starts up the leak tester in calibration mode, then applies a constant amount of volumetric change (ΔV) to the WORK measurement system during detection time. The differential pressure which results (ΔP) is detected, and the volume equivalent (Ve) value of the measurement system is automatically calculated. The measured Ve can be used to express it in a unit of leak rate (e.g. mL/min).
| Ve:Volume equivalent (mL) ΔV:Amount of volumetric change (mL) ΔP:Differential pressure (Pa) P:Test pressure (kPa) |
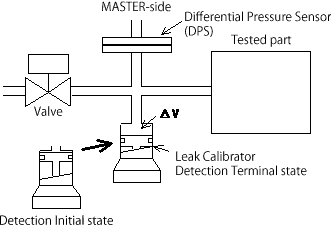
<※COMMENT>
Outline of regarding auto leak calibrators
- 1 Calculates and displays the volume equivalent of WORK’s side.
- 2 This step to calculate the volume equivalent is also the step which calibrates the leak testing device. (K check)
- 3 Displays, not only as differential pressure, but as leak rate.
- 4 Has the ability to make pass or fail judgments based on the leak rate, not only differential pressure.
2 Choosing a leak calibrator
A leak calibrator finds the amount of volumetric change using the following equation, and selects the format depending on the test conditions. There are automatically operating leak calibrators and manually operated calibrators (types LC and QLC).
| ΔV:Amount of volumetric change (mL) Ve :Presumed volume equivalent (mL) P :Test pressure (kPa) ΔP:Differential pressure (Pa) Maximum 1000 Pa | |
| Auto leak calibrator Built into the leak tester and is fully automated. | |
| Type LC Turn the dial during detection to cause volumetric change. May not be operable depending on the test pressure. | |
| Type QLCVolumetric change of any amount can be caused with just a touch of a button. Its easy operation makes it perfect for daily checks. It can be operated while the test pressure is being applied. |
Choose the leak calibrator whose value is the closest to the full scale ΔV found when ΔP is 1000 Pa (may be above or below the full scale).
※F.S. = full scale
| Test conditions | Auto leak calibrator | Manual leak calibrator | |||||
|---|---|---|---|---|---|---|---|
| Type LC | Type QLC | ||||||
| WORK volume | Test pressure | Model | F.S.* | Model | F.S.* | Model | F.S.* |
| Small | Medium/High | ALC-05 | 0.5mL | - | - | QLC-0021 | 0.2mL |
| Small/Medium | Low/Medium/High | ALC-1 | 1mL | LC-12 | 1mL | QLC-0101 | 1mL |
| Small/Medium/Large | Low/Medium | - | - | LC-22 | 2mL | - | - |
| Medium/Large | Low/Medium | ALC-4 | 4mL | LC-42 | 4mL | QLC-0401 | 4mL |
| Large (option) | Low/Medium | ALC-10 | 10mL | - | - | QLC-1001 | 10mL |
3. K(Ve) calibration using a leak MASTER
A feature which applies a constant leak to the WORK measurement system to find the volume equivalent.
Connect a leak MASTER to a calibration port and start it up in calibration mode to calculate and display K(Ve). This feature is available on LS-1881, LS-R900, and LS-R700. LS-1866 uses the leak MASTER to calculate the flow coefficient and converts the differential pressure into leak rate.


